Question

Two disks of polaroid are aligned so that they polarize light in the same plane. Calculate the angle through which one sheet needs to be turned.

Answer 1

Answer: The unpolarized light's intensity is reduced by the factor of two when it passes through the polaroid and becomes linearly polarized in the plane of the Polaroid. When the polarized light passes through the polaroid with the plane of polarization at an angle
`\theta` with respect to the polarization plane of the incoming light, the light's intensity is reduced by the factor of
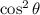`\cos^2\theta` (this is the Law of Malus).

Explanation: Let us say we have a beam of unpolarized light of intensity
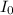`I_0` that passes through two parallel Polaroid discs with the angle of
`\theta` between their planes of polarization. We are asked to find
`\theta` such that the intensity of the outgoing beam is
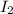`I_2`. To solve this we follow the steps below:

Step 1. It is known that when the unpolarized light passes through a polaroid its intensity is reduced by the factor of two, meaning that the intensity of the beam passing through the first polaroid is

`I_1=(I_0)/(2).`

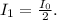
This beam also becomes polarized in the plane of the first polaroid.

Step 2. Now the polarized beam hits the surface of the second polaroid whose polarization plane is at an angle
`\theta` with respect to the plane of the polarization of the beam. After passing through the polaroid, the beam remains polarized but in the plane of the second polaroid and its intensity is reduced, according to the Law of Malus, by the factor of
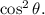`\cos^2\theta.` This yields
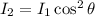`I_2=I_1\cos^2\theta`. Substituting from the previous step we get

`I_2=(I_0)/(2)\cos^2\theta`

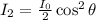
yielding

`(2I_2)/(I_0)=\cos^2\theta`

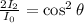
and finally,

`\theta=\arccos\sqrt{(2I_2)/(I_0)}`