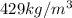1 Answer

Quealegriamasalegre · Answer 1 · 2021-10-11T06:29:33+0000

To solve this problem we will apply the principle of buoyancy of Archimedes and the relationship given between density, mass and volume.

By balancing forces, the force of the weight must be counteracted by the buoyancy force, therefore

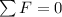
$\sum F = 0$

F_b -W = 0

F_b = W

F_b = mg

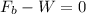
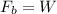
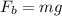
Here,

m = mass

g =Gravitational energy

The buoyancy force corresponds to that exerted by water, while the mass given there is that of the object, therefore

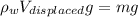
$\rho_w V_(displaced) g = mg$

Remember the expression for which you can determine the relationship between mass, volume and density, in which

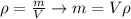
$\rho = (m)/(V) \rightarrow m = V\rho$

In this case the density would be that of the object, replacing

$\rho_w V_(displaced) g = V\rho g$

Since the displaced volume of water is 0.429 we will have to

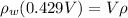
$\rho_w (0.429V) = V \rho$

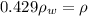
$0.429\rho_w= \rho$

The density of water under normal conditions is
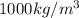1000kg / m ^ 3 , so

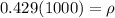
$0.429(1000) = \rho$

$\rho = 429kg/m^3$

The density of the object is
429kg / m ^ 3

Please log in or register to add a comment.