Question

The acceleration due to gravity on some planet is 18.9 m/s2. If you weigh 1081 N on Earth, how much would you weigh on that planet?V

Answer 1

The acceleration is
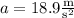`a=18.9\mathrm{(m)/(s^2)}` and force is
`F=1081\mathrm{N}`.

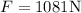Newtons second law of motion that when an object is accelerating its force (weight in current context) can be calculated using his mass:

`F=ma`

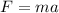
If we express mass:

`m=(F)/(a)`

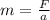
We first need to find mass since mass won't change no matter the acceleration.

If we know that acceleration on earth equals
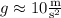`g\approx10\mathrm{(m)/(s^2)}` then we can calculate our mass:

`m=(F)/(g)=\frac{1081\mathrm{N}}{10\mathrm{(m)/(s^2)}}=108.1\mathrm{kg}`

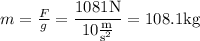
Then we use this mass and calculate our weight on a different planet:

`F=ma=108.1\mathrm{kg}\cdot18.9\mathrm{(m)/(s^2)}=\boxed{2043.09\mathrm{N}}`

Hope this helps.

Edit one:

Also note that I have rounded
`g` to
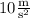`10\mathrm{(m)/(s^2)}` if wish to get more precise results use
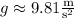`g\approx9.81\mathrm{(m)/(s^2)}`.