Question

The sum of the interior angle measures of ABC is 180 proof

Answer 1

The Proof for, The sum of the interior angle measures of ΔABC is 180° is below.

Explanation:

Given:

Draw a ΔABC ,

Construction:

Draw PQ Parallel to Side of triangle BC,

PQ || BC

To Prove:

The sum of the interior angle measures of ABC is 180.

`\angle ABC+\angle BAC+\angle ACB=180\°`

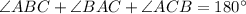
Proof:

P - A - Q is a Straight Line, m∠PAQ = 180° ...... Straight Angle

∴
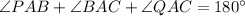`\angle PAB+\angle BAC+\angle QAC=180\°` ....Angle Addition Postulate...........( 1 )

PQ || BC

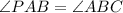`\angle PAB=\angle ABC` .....Alternate Angle Postulate as PQ || BC...( 2 )

Similarly,

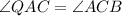`\angle QAC=\angle ACB` .....Alternate Angle Postulate as PQ || BC...( 3 )

Now by Substituting ∠PAB and ∠QAC in ( 1 ) and ,Transitive Property we get From ( 1 ) , ( 2 ) and ( 3 )

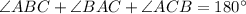`\angle ABC+\angle BAC+\angle ACB=180\°` ...Proved

i.e The sum of the interior angle measures of Δ ABC is 180°