Question

A pole, 4m high, as shown in figure 2, stands, vertically, on

level ground. If the sun is at an angle of 30° to the horizontal,
how long is the shadow.

Use the tangent ratio to solve the problem.​

Answer 1

Answer: 6.92 m

Explanation:

Assuming the image is the attached below, we have a right triangle, where one of the legs is the 4 m high pole and the other leg the shadow.

Now, the tangent function is defined as:

`tan \theta=(Opposite-leg)/(Adjacent-leg)`

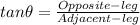
Since the Sun is at an angle
`\theta=30\°` and the adjacent leg is the shadow
`s` we have:

`tan(30\°)=(4 m)/(s)`

Isolating
`s`:

`s=(4 m)/(tan(30\°))`

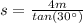
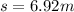`s=6.92 m` This is the length of the shadow