Answer:
The value of the given expression is

Explanation:
Given that
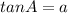
To find the value of

Let us find the value of the expression
 :
:
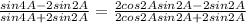 ( by using the formula
( by using the formula
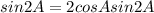 here A=2A)
here A=2A)
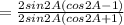
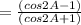
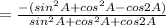 (using
(using
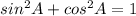 here A=2A)
here A=2A)
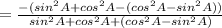 (using
(using
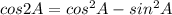 here A=2A)
here A=2A)
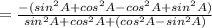

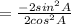
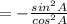
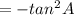 ( using here A=2A )
( using here A=2A )
(since tanA=a given )
Therefore
