Answer:
N(p) = -120p + 850
Explanation:
Let the number of lattes sold by the coffee shop be N
and p be the price of each Lattes
The number of lattes (N) sold by a coffee shop is linearly related to the sale price (p).
Now,Let the linear relationship be
N(p) = mp+c, -------------------(1)
where
m is the slope
c is an arbitrary real number.
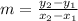 --------------------(2)
--------------------(2)
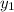 = number of lattes sold currently = 490
= number of lattes sold currently = 490
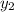 = number of lattes sold previously = 490 - 30
= number of lattes sold previously = 490 - 30
 = cost of lattes sold currently = 3.00
= cost of lattes sold currently = 3.00
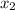 = cost of lattes sold previously = 3.00+0.25
= cost of lattes sold previously = 3.00+0.25
Substituting the values in eq(2)
Now, m =
![([(490-30)-490])/([(3.00+0.25)-3.00])](https://img.qammunity.org/2021/formulas/mathematics/high-school/ms6l8us6yityq3ru7we0ncllxa1tdljxdo.png)
m =
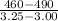
m =
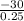
m = -120.
On substituting m = -160 eq(1), we get
N(p) = -120p+c.
Further, on substituting N = 430 and p = 2.75 , we get
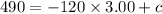
490 = -360 + c
490+360 = c
c = 850
Thus
N(p) = -120p + 850