Answer:
Δ SJX ~ Δ LXP
ΔSJX ~ ΔLXP ….{Angle-Angle Similarity Postulate}
Explanation:
Given:
∠XSJ ≅ ∠XLP = 73°
∠SXJ = 54°
∠LXP = 53°
To Prove:
ΔSJX ~ ΔLXP
Proof:
Triangle sum property:
In a Triangle sum of the measures of all the angles of a triangle is 180°.
In ΔLPX,
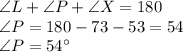
∴ ∠SXJ ≅ ∠LPX = 54° ..........Transitive Property ..( 1 )
In Δ SJX and Δ LXP
∠XSJ ≅ ∠XLP …………..{ measure of each angle is 73° given }
∠SXJ ≅ ∠LPX = 54° ……….....{From ( 1 ) above}
ΔSJX ~ ΔLXP ….{Angle-Angle Similarity Postulate} ..Proved