Answer:
22.404 inches of the wire should be used for square
17.596 inches of the wire should be used for the circle.
Explanation:
We're forming one square and one circle from a 40 inch long wire.
Hence we know that the sum of the perimeters(P) of the two shapes will be 40.
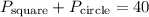
let,
 be the side-length of the square
be the side-length of the square
 be the radius of the circle.
be the radius of the circle.
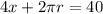
It is also stated that the combined area of both shapes should be minimum.
Hence, we can formulate the sum of the areas(A) similarly to how we did for perimeters.
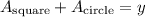
 is the combined area
is the combined area
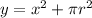
this is a function y(x,r). In order to find the minimum combined area, we need to differentiate the function. But first we need to replace one of the variables 'x' or 'r' so that the function 'y' only has one variable.
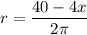
we can substitute this in y
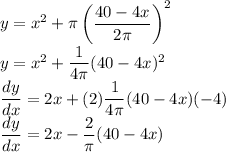
now substitute

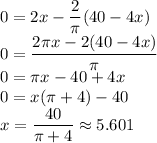
we can use this to find 'r'.
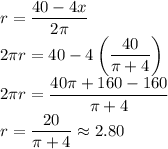
these are the dimensions of the two shapes for which the combined areas will be minimum.
we can find how much of the wire segments will be used for each of the shapes.
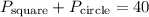
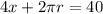
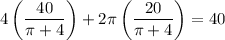
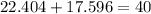
22.404 inches of the wire should be used for square
17.596 inches of the wire should be used for the circle.