Answer:
The sample size should be approximately 167.
Explanation:
We are given the following in the question
Sample size, n = 10
Confidence level = 99%
Significance level = 0.01
Standard deviation, σ = 50
Error = 10
Formula:

Ptting values, we get,
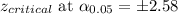

The sample size should be approximately 167.