Answer:
Therefore,
The value of arcsin(0) is 0° or 180° or 360° or 540° or n×180° so on.
Explanation:
To Find:
arcsin(0) in degrees =?
Solution:
' arcsin ' a mathematical function that is the inverse of the sine function.
Therefore, arcsin (x) is called the inverse sine function.
It is the angle whose sine is the number x.
So here we require angle in degree for which the Sine is '0'.
It is also written as
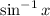
We Know that
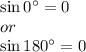
Therefore,
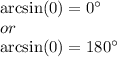
Also integer multiples of 180° will have
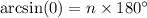
Where n = integer
Therefore for '0' we can have 2×180° , 3×180° , 4×180°....and so on i.e 360° , 540° and so on
Therefore,
The value of arcsin(0) is 0° or 180° or 360° or 540° or n×180° so on.