Question

A bee flies at 20 feet per second directly to a flowerbed from its hive. The bee stays at three flowerbed for 15 minutes, then flies directly back from the hive for a total of 20 minutes.

Answer 1

Question is Incomplete, Complete question is given below;

A bee flies at 20 feet per second directly to a flowerbed from its hive. The bee stays at the flowerbed for 15 minutes, then flies directly back to the hive at 12 feet per second. It is away from the hive for a total of 20 minutes. a. What equation can you use to find the distance of the flowerbed from the hive?

b. How far is the flowerbed from the hive?

Answer:

a) The equation representing the distance of the flowerbed from the hive
`(x)/(20)+900+(x)/(12) =1200`.

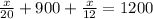b) The Flower bed is 2250 feet away from the hive.

Explanation:

Speed from hive to flower bed = 20 ft/s

Time at flower bed = 15 minutes.

Now we will convert mins in seconds.

1 min = 60 seconds.

15 min = number of seconds in 15 mins.

By Using Unitary method we get;

number of seconds in 15 mins =
`15*60 = 900 \ seconds`

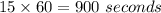
Time at flower bed = 900 seconds

Also Given:

Speed from flower bed to hive = 12 ft/s

Total time = 20 minutes.

Now we will convert mins in seconds.

1 min = 60 seconds.

20 min = number of seconds in 20 mins.

By Using Unitary method we get;

number of seconds in 20 mins =
`20*60 = 1200 \ seconds`

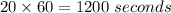
Total time = 1200 seconds.

We need to find:

a) the equation representing the distance of the flowerbed from the hive

b) to find the distance of the flowerbed from the hive.

Solution:

Solving for part a.

Let the distance of the flowerbed from the hive be 'x'.

Now we know that;

Time is equal to Distance divide by speed.

Time from hive to flower bed =
`\frac{\textrm{distance of the flowerbed from the hive}}{\textrm{speed of bee from hive to flower bed}}`

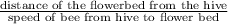
Time from hive to flower bed =
`(x)/(20) \ seconds`

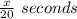
Also Time from flower bed to =
`\frac{\textrm{distance of the flowerbed from the hive}}{\textrm{speed of bee from flower bed to hive}}`

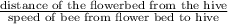
Time from hive to flower bed =
`(x)/(12) \ seconds`

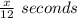
Now we can say that:

Total Time taken is equal to Time from hive to flower bed plus Time at flower bed plus Time from hive to flower bed.

framing in equation form we get;

`(x)/(20)+900+(x)/(12) =1200`

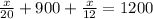
Hence the equation representing the distance of the flowerbed from the hive
`(x)/(20)+900+(x)/(12) =1200`.

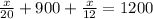Solving for part b.

Now to find the distance of the flowerbed from the hive we solve the above equation.

By taking LCM we get;

LCM of 20 and 12 is 60

`(xtimes3)/(20*3)+(900*60)/(60)+(x*5)/(12* 5) =1200\\\\(3x)/(60)+(54000)/(60)+(5x)/(60) =1200\\\\(3x+54000+5x)/(60)=1200\\\\3x+54000+5x =1200*60\\\\8x+54000 = 72000\\\\8x =72000-54000\\\\8x =18000\\\\x =(18000)/(8) =2250\ ft`

Hence the Flower bed is 2250 feet away from the hive.