Answer:
about 19 years
Step-by-step explanation:
The formula for determining the total amount at the end of an investment, with interest compounded annually is,
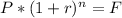
where P is the amount invested,
r is the rate of return
n is the number of years
and F is the total amount at the end of the investment.
Therefore, for $1 invested today to triple ($3) at 6% annual rate,
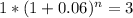
=
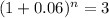
By interpolation, the value of n that satisfies the equation is 18.9.
Therefore, the investment will triple in 18.9 years.