Question

There are 10 students in a class. The teacher chooses 2 students to go to the

library. The order in which they are chosen does not matter. How many ways
are there to choose the students?
ОА. 100
оB. 720
ОC. 45
OD. 90

Answer 1

The number of ways in which students were chosen is 45 ways .

Explanation:

Given as :

The number of students in a class = x = 10

The number of students chosen by teacher to go to library = y = 2

Let The number of ways in which students were chosen = n ways

Now, According to question

So, number of ways in which students were chosen =
`_(y)^(x)\textrm{C}`

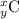
Or, n ways =
`_(2)^(10)\textrm{C}`

Or, n =
`(\L 10)/(\L( 10-2)* \L 2)`

Or, n =
`(\L 10)/(\L8* \L 2)`

Or, n =
`(10* 9* \L 8)/(\L 8* \L 2)`

Or, n =
`(10* 9)/(2* 1)`

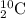
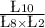
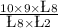
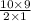
Or, n = 5 × 9

i.e n = 45

So, The number of ways in which students were chosen = n = 45 ways

Hence,The number of ways in which students were chosen is 45 ways . Answer