Question

Differentiating a Logarithmic Function in Exercise, find the derivative of the function. See Examples 1, 2, 3, and 4.

y = ln x/x^2

Answer 1

`(dy)/(dx)=(x(1-2lnx))/(x^(4))`

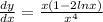
Explanation:

To solve the question we refresh our knowledge of the quotient rule.

For a function f(x) express as a ratio of another functions u(x) and v(x) i.e

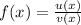`f(x)=(u(x))/(v(x))\\`, the derivative is express as

`(df(x))/(dx)=(v(x)(du(x))/(dx)-u(x)(dv(x))/(dx))/(v(x)^(2) )`

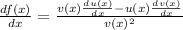
from
`y=lnx/x^(2)`

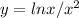
we assign u(x)=lnx and v(x)=x^2

and the derivatives

`(du(x))/(dx)=(1)/(x)\\(dv(x))/(dx)=2x\\`.

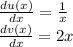Note the expression used in determining the derivative of the logarithm function.it was obtain from the general expression of logarithm derivative i.e
`y=lnx\\(dy)/(dx)=(1)/(x)`

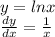
If we substitute values into the quotient expression we arrive at

`(dy)/(dx)=((x^(2)*(1)/(x))-(2x*lnx))/(x^(4))\\(dy)/(dx)=(x-2xlnx)/(x^(4))\\(dy)/(dx)=(x(1-2lnx))/(x^(4))`