Answer:
(a) 8.15
(b) 12.92
Explanation:
Given: P = $3000, r = 0.085
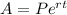
Where
A is the Amount
P is the Principal
r is the rate
t is the time
(a) For the amount to double, A = 2 × P
A = 2 × $3000
A = $6000
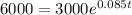
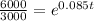
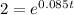
Take
 of both sides
of both sides
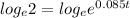
But

∴

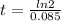
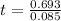
t = 8.15
(b) For the amount to double, A = 3 × P
A = 3 × $3000
A = $9000
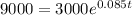
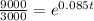
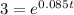
Take
 of both sides
of both sides
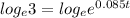
But

∴
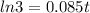
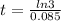
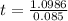
t = 12.92