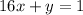Question

Finding an Equation of a tangent Line in Exercise, find an equation of the tangent line to the graph of the function at the given point.

y = (e4x - 2)2, (0 , 1)

Answer 1

`16x+y=1`

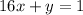
Explanation:

We are given that

`y=(e^(4x)-2)^2`

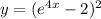
Point (0,1)

We have to find the equation of tangent line to the given graph.

Differentiate w.r.t x

`(dy)/(dx)=2(e^(4x)-2)(e^(4x)* 4)`

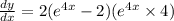
By using formula

`(d(x^n))/(dx)=nx^(n-1)`

`(d(e^x))/(dx)=e^x`

`m=(dy)/(dx)=8e^(4x)(e^(4x)-2)`

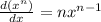
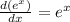
Substitute x=0

`m=(dy)/(dx)=8(-2)=-16`

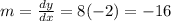
Slope-point form:

`y-y_1=m(x-x_1)`

`x_1=0,y_1=1`

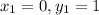
Using the formula

`y-1=-16(x-0)=-16x`

`16x+y=1`

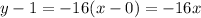
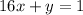
Hence, the equation of tangent line to the function at point (0,1)

`16x+y=1`