Question

A function
`f(x)=√(x)` is transformed into the function
`g(x)=3√(x-2) +5`

Name the 3 transformations that occurred and describe the general shape of g(x). When describing the shape, you have the option of including a picture of its graph.

PLEASE HELP!!

Answer 1

vertical stretch by a factor of 3, horizontal shift of 2 units to the right, and a vertical shift 5 units up.

Explanation:

The parent function is

`f(x) = √(x)`

The transformed function is

`g(x) = 3 √(x - 2) + 5`

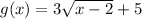
This transformation is of the form

`g(x) = a \cdot \: f(x + b) + c`

where a=3 is the vertical stretch by a factor of 3.

b=-2 is a horizontal shift to the right by 2 units.

c=5 is a vertical upward shift by 5 units.