Answer:
False
Explanation:
the dot product between two vectors A and B is:
A·B=AB

where
 is the angle between the vectors, if they are parallel, this angle is zero. so
is the angle between the vectors, if they are parallel, this angle is zero. so
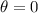
and so the dot product is:
A·B =AB

and since

the dot product is equal to
A·B=AB
The dot product of parallel vectors is NOT zero