Question

A 14 kg tennis ball moves at a velocity of 11 m/s. The ball is struck by a racket, causing it to rebound in the opposite direction at a speed of 5 m/s. What is the change in the ball’s momentum? Report the number of kg‧m/s with the appropriate sign.

Answer 1

To solve this problem we will apply the concepts related to the momentum.

This is defined as the product between the change in velocity and the mass of the object, that is

`p = m\Delta v`

`p = m (v_f-v_i)`

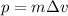
Where,

m = mass

`v_f =` Final velocity

`v_i =` Initial velocity

Our values are given as,

m = 14kg

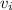`v_i`= 11m/s

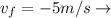`v_f= - 5m/s \rightarrow` the negative Symbol implies that the direction is opposite to the initial one and therefore there is also a change in the sense of magnitude

`p = (14)(-5-11)`

`p = 14(-16)`

`p = -224 kg \cdot m/s`

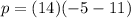
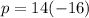
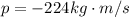
The negative symbol indicates that the momentum has a direction opposite to that of the initial velocity. Or failing that, it has the same direction of the final speed