Question

According to a recent​ survey, the population distribution of number of years of education for​ self-employed individuals in a certain region has a mean of 15.1 and a standard deviation of 4.8.

a. Identify the random variable X whose distribution is described here.
b. Find the mean and the standard deviation of the sampling distribution of x overbar for a random sample of size 36. Interpret them.
c. Repeat​ (b) for nequals144. Describe the effect of increasing n.

Answer 1

a) X that is a distribution of number of years of education for​ self-employed individuals.

b) Mean = 15.1, Standard Deviation = 0.8

c) Mean = 15.1, Standard Deviation = 0.4

Explanation:

We are given the following in the question:

The population distribution of number of years of education for​ self-employed individuals in a certain region has a mean of 15.1 and a standard deviation of 4.8

a) The random variable is X that is a distribution of number of years of education for​ self-employed individuals.

b) According to central limit theorem, as the sample size increases the distribution of means approaches a normal distribution.

Thus,
`\bar{x}` has a normal distribution with

`\text{Mean} = 15.1\\\text{Srtandard Deviation} = \displaystyle(\sigma)/(√(36)) = (4.8)/(√(36)) = 0.8`

c) n = 144

`\bar{x}` has a normal distribution with

`\text{Mean} = 15.1\\\text{Srtandard Deviation} = \displaystyle(\sigma)/(√(n)) = (4.8)/(√(144)) = 0.4`

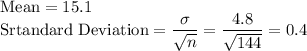
By increasing n, the standard deviation for distribution of mean reduced by one half. Therefore, we see that quadrupling the sample size will reduce the standard deviation by one half.