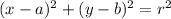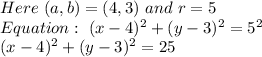Question

What is the equation of a circle with diameter AB that has endpoints A(0, 0) and B(8, 6)?

A. (x − 4)2 + (y − 3)2 = 25

B. (x − 3)2 + (y − 4)2 = 5

C. (x − 4)2 + (y − 3)2 = 5

D.(x − 3)2 + (y − 4)2 = 25

Answer 1

A.
`(x-4)^2+(y-3)^2=25`

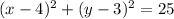
Explanation:

Radius:

The length of diameter
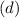`(d)` is the distance between A and B.

`d=√((8-0)^2+(6-0)^2)=√(64+36)=√(100)=10\\\\radius(r)=(d)/(2)=(10)/(2)=5`

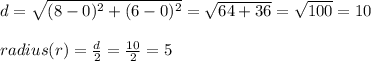
Centre:

Since A and B are end points of the diameter, centre is the mid point of these two. Let
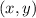`(x,y)` be the centre of the circle.

`x=(8+0)/(2)=4\\\\y=(6+0)/(2)=3\\\\`

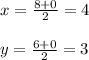
Centre is
`(4,3)`

Equation of circle:

If
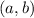`(a,b)` is the centre of the circle and
`r` be the radius. Equation of circle is given by:

`(x-a)^2+(y-b)^2=r^2`

`Here\ (a,b)=(4,3)\ and\ r=5\\Equation:\ (x-4)^2+(y-3)^2=5^2\\(x-4)^2+(y-3)^2=25`