Question

[calculus] the distance from the point (6, -4) to the circle
`x^2+y^2-8y=0` is

(A)
`√(10)` (B) 4 (C) 6 (D) 10 (E) none of these

Answer 1

Option C.

The distance from the point to the circle is 6 units

Explanation:

we know that

The distance between the circle and the point will be the difference of the distance of the point from the center of circle and the radius of the circle

step 1

Find the center and radius of the circle

we have

`x^(2) +y^(2)-8y=0`

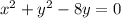
Convert to radius center form

Complete the square

`x^(2) +(y^(2)-8y+16)=0+16`

Rewrite as perfect squares

`x^(2) +(y-4)^(2)=16`

so

The center is the point (0,4)

The radius is

`r^(2)=16\\r=4\ units`

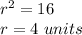
step 2

Find the distance of the point from the center of circle

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

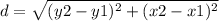
we have

(6,-4) and (0,4)

substitute the values

`d=\sqrt{(4+4)^(2)+(0-6)^(2)}`

`d=\sqrt{(8)^(2)+(-6)^(2)}`

`d=√(100)\ units`

`d=10\ units`

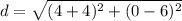
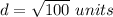
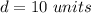
step 3

Find the difference of the distance of the point from the center of circle and the radius of the circle

`10\ units-4\ units=6\ units`

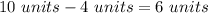
therefore

The distance from the point to the circle is 6 units

see the attached figure to better understand the problem