Question

If 3 workers can paint a room in 2 hours, then approximately how long does it take 4 workers to paint the same room? Assume the time needed to paint the room is inversely proportional to the number of workers.

Answer 1

It takes 1.5 hours for 4 workers to paint the same room

Solution:

Given that 3 workers can paint a room in 2 hours

To find: Time taken for 4 workers to paint the same room

Assume the time needed to paint the room is inversely proportional to the number of worker

`time $ \propto \frac{1}{\text { number of workers }}\\\\time =k * \frac{1}{\text { number of workers }}`

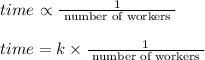
Where, "k" is the constant of proportionality

3 workers can paint a room in 2 hours

Substitute number of workers = 3 and time = 2 hours

`time =k * \frac{1}{\text { number of workers }}\\\\2 = k * (1)/(3)\\\\k = 6`

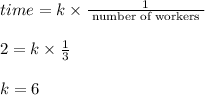
Therefore,

`\text {time}=6 * \frac{1}{\text { number of workers }}`

To find time taken for 4 workers to paint the same room, substitute number of workers = 4 in above expression

`time = 6 * (1)/(4) = 1.5`

Thus it takes 1.5 hours for 4 workers to paint the same room