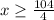Question

The length of a rectangular boat storage shed must be 4 meters more than the width, and the perimeter must be at least 112 meters. What is the range of values for the width?

Answer 1

The width of the rectangular shed can be 26, 27, 28, ........

Explanation:

Let the width of the rectangular shed be, W = x

Then the length of the rectangular shed will be

L = 4 + width

L = 4+x

We know that the perimeter of the rectangle is 2W+2L

Also it is given that the perimeter of the rectangular must be at least 112 meters

2W+2L >= 112

Substituting the values

`2x+4x+8 \geq 112`

`2x+8+2x \geq 112`

`4x+8 \geq 112`

`4x \geq 112-8`

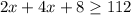
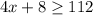
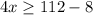
4x>104

`x \geq (104)/(4)`

`x \geq 26`