Question

A: {71,73,79,83,87} B:{57,59,61,67}

If one number is selected at random from set A, and one number is selected at random from set B, what is the probability that both numbers are prime?

Answer 1

`(3)/(5)`.

Explanation:

We have been given two sets as A: {71,73,79,83,87} B:{57,59,61,67}. We are asked to find the probability that both numbers are prime, if one number is selected at random from set A, and one number is selected at random from set B.

We can see that in set A, there is only one non-prime number that is 87 as it is divisible by 3.

So there are 4 prime number in set A and total numbers are 5.

`P(\text{Prime number from A})=(4)/(5)`

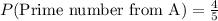
We can see that in set B, there is only one non-prime number that is 57 as it is divisible by 3.

So there are 3 prime number in set B and total numbers are 4.

`P(\text{Prime number from B})=(3)/(4)`

Now, we will multiply both probabilities to find the probability that both numbers are prime. We are multiplying probabilities because both events are independent.

`P(\text{Prime number from A and B})=(4)/(5)* (3)/(4)`

`P(\text{Prime number from A and B})=(1)/(5)* (3)/(1)`

`P(\text{Prime number from A and B})=(3)/(5)`

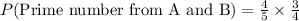
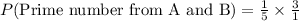
Therefore, the probability that both numbers are prime would be
`(3)/(5)`.