Question

There are 40 people at Drake’s sweet sixteen party. Drake’s only rule is that everyone in the party must meet and shake each other’s hand. If all40 guest shake each other’s hand once and only once.

1. How many handshakes are there altogether?
2. How did you get your solution?
3. What’s the quickest way to figure this out if Drake invited 1,000 people?

Answer 1

Given:

Total Number of people at the Drake’s sweet sixteen party = 40

Everyone in the party must meet and shake each other’s hand

1) How many handshakes are there altogether?

There was total of 780 handshakes in the party.

2. How did you get your solution:

If you have 7 people, person seven shakes six other hands, person

six shakes five other hands, person five shakes fours other hands,

person ,four shakes three other hands, person three shakes two other hands, and person two shakes one hand. Another way to see it is,

Person 7 +person 6 + person 5 + person 4 +person 3 + person 2 + person 1

= 6+5+ 4+3+2+1

=>21

so similairly in case of 40 persons

= 39+38+37+36+37+36+35+34 .................+3+2+1

=>780

3) What’s the quickest way to figure this out if Drake invited 1,000 people?

People at Party Number of Handshakes

2 1

3 1 + 2 = 3

4 1 + 2 + 3 = 6

5 1 + 2 + 3 + 4 = 10

6 1 + 2 + 3 + 4 + 5 = 15

.

.

.

n 1 + 2 + ...+ (n-1) = --------
`(n(n-1))/(2)`

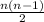
Hence for n persons the number of handshakes will be
`(n(n-1))/(2)`

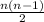
So for 1000 persons the number of handshakes will be

=>
`(1000(1000-1))/(2)`

=>
`(1000(999))/(2)`

=>
`(999000)/(2)`

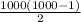
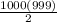
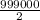
=>499,500