Question

A rope, assumed massless is stretched horizontally between

twosupports that are 3.44 m apart. When an object of weight 3160 N
ishung at the center of the rope, the rope is observed to sag by
35.0cm. What is the tension of the rope?

Answer 1

7900N

Step-by-step explanation:

35cm = 0.35m

When the object is at the rope center, its position at 3.44 / 2 =1.72m. We can find out the angle that the sagged rope makes with the horizontal

`\theta = tan^(-1)(0.35/1.72) = 11.5 degree`

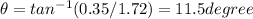
this means the rope makes with the vertical an angel of

90 - 11.5= = 78.5 degree

The tension of the 2 ropes, T, should balance with the object weight

`2T*cos(78.5) = 3160`

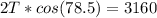
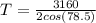`T = (3160)/(2cos(78.5))` = \frac{3160}{2*0.2} = 7900N[/tex]