Answer:
Since, the six main trigonometric functions are:
- Sine (sin)
- Cosine (cos)
- Tangent (tan)
- Secant (sec)
- Cosecant (csc)
- Cotangent (cot)
If we have the exact value of any one trigonometric function for a degree then we can find the other function for the same degree as follow.
sin 300° = sin (-60 + 360)° = sin (-60) = - sin 60 =
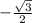
cos 300° = cos (-60 + 360)° = cos (-60) = cos 60 =

tan 300° =
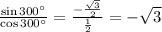
cot 300° =

sec 300° =
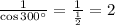
csc 300° =
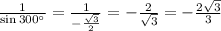
Note : sin (-x) = -sin x and cos (-x) = cos x