Question

A boat travels at the rate of 7mph in still water and travels at 12 miles downstream in the same time it travels 8 miles upstream. What is the rate if the current?

Answer 1

Explanation:

It's easiest to make a table to keep your stuff organized. I find in my classroom that it helps the students to see where the values go and to better determine what the equations are that result from the table. Sometimes it helps to draw pictures here, but not the case here, thankfully (cuz I can't draw you a picture here!)

We will have DS (downstream) and US (upstream) as our 2 trips. Distance = rate times time. So here's our table, filling in the fact that the distance DS is 12 and distance US is 8:

d = r x t

DS 12

US 8

The first sentence says that the rate of the boat in still water is 7, but we are looking for the rate of the water, r. As you can see from the numbers in our table, DS moves the boat along quicker, and that is because the current is pushing it, or adding to the speed of the boat. We represent that as r + 7, which is the rate of the current added to the speed that the boat can travel with only its engine moving it in the water. US the boat moves slower since the current of 7 mph is taking away from its speed in still water. We represent that as r - 7. Filling that into our table:

d = r x t

DS 12 r + 7

US 8 r - 7

Now for the last bit of info. It says that the boat travels 12 miles DS IN THE SAME AMOUNT OF TIME that it travels US. That means that, regardless of how long it takes to travel these distances, they are both the same. Do we know what that length of time is? No, we don't, so we will call it "t":

d = r x t

DS 12 r + 7 t

US 8 r - 7 t

Using the formula for distance, d = rt, we can write the 2 equations that result in each row.

DS

12 = (r + 7)t

US

8 = (r - 7)t

Because the times are the same, we can now solve each equation for t and set them equal to each other. If

12 = (r +7)t, then

`t=(12)/(r+7)`

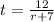
If

8 = (r - 7)t, then

`t=(8)/(r-7)`

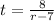
By the transitive property of equality, if a = b, and b = c, then a = c. That means to us that if DS has a time that is equal to the time for US, then t = t and

`(12)/(r+7) =(8)/(r-7)`

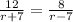
Cross mutiply to solve for r:

12(r - 7) = 8(r + 7) and

12r - 84 = 8r + 56 and

4r = 140 so

r = 35

The rate of the current is 35 mph.