Question

A sample of radiosodium () has a half-life of 15 hr. If the sampleâs activity is 100 millicuries after 24 hr, approximately what must its original activity have been?

Answer 1

Answer : The original activity will be, 303 millicuries.

Explanation :

Half-life = 15 hr

First we have to calculate the rate constant, we use the formula :

`k=(0.693)/(t_(1/2))`

`k=(0.693)/(15hr)`

`k=4.62* 10^(-2)\text{ hr}^(-1)`

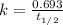
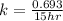
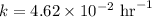
Now we have to calculate the time passed.

Expression for rate law for first order kinetics is given by:

`t=(2.303)/(k)\log(a)/(a-x)`

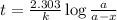
where,

k = rate constant =
`4.62* 10^(-2)\text{ hr}^(-1)`

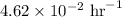
t = time passed by the sample = 24 hr

a = initial amount of the reactant = ?

a - x = amount left after decay process = 100 millicuries

Now put all the given values in above equation, we get

`24=(2.303)/(4.62* 10^(-2))\log(a)/(100)`

`a=302.97\text{ millicuries}\approx 303\text{ millicuries}`

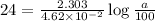
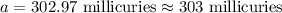
Therefore, the original activity will be, 303 millicuries.