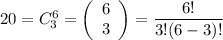Question

Which of the following demonstrates how the 20 is calculated using the
combination pattern?

Answer 1

D

Explanation:

The diagram shows Pascal's triangle. Pascal's triangle is a triangular array of the binomial coefficients.

The entry in the
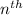`n^(th)` row (start counting rows from 0) and
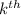`k^(th)` column (start counting columns from 0) of Pascal's triangle is denoted by

`C^n_k=\left(\begin{array}{c}n\\ k\end{array}\right)`

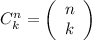
Coefficient 20 stands in 6th row, then n = 6 and in 3rd column, so k = 3.

Hence,

`20=C^6_3=\left(\begin{array}{c}6\\ 3\end{array}\right)=(6!)/(3!(6-3)!)`