Option B
The choice has a value that is closest to the value of the following expression 17/12 - 49/40 is
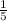
Solution:
Given that we have to find the value that is closest to the value of following expression

Let us take L.C.M of denominators and solve the sum
L.C.M of 12 and 40
List all prime factors for each number
prime factorization of 12 = 2 x 2 x 3
prime factorization of 40 = 2 x 2 x 2 x 5
For each prime factor, find where it occurs most often as a factor and write it that many times in a new list.
The new superset list is
2, 2, 2, 3, 5
Multiply these factors together to find the LCM.
LCM = 2 x 2 x 2 x 3 x 5 = 120
Thus the given expression becomes:
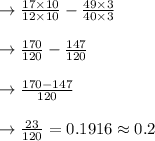
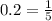
Thus correct answer is option B