Question

A 1.6-kg block is attached to the end of a 2.0-m string to form a pendulum. The pendulum is released from rest when the string is horizontal. At the lowest point of its swing when it is moving horizontally, the block is hit by a 10-g bullet moving horizontally in the opposite direction. The bullet remains in the block and causes the block to come to rest at the low point of its swing. What was the magnitude of the bullet's velocity just before hitting the block?

Answer 1

1002.2688 m/s

Step-by-step explanation:

g = Acceleration due to gravity = 9.81 m/s²

h = The length of a string = 2 m

m = Mass of block = 1.6 kg

`m_2` = Mass of bullet = 0.01 kg

Here, the potential energy of the fall will balance the kinetic energy of the bullet

`mgh=(1)/(2)mv^2\\\Rightarrow v=√(2gh)\\\Rightarrow v=√(2* 9.81* 2)\\\Rightarrow v=6.26418\ m/s`

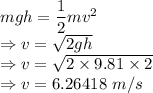
Velocity of block is 6.26418 m/s

As the momentum of system is conserved we have

`mv=m_2u\\\Rightarrow u=(mv)/(m_2)\\\Rightarrow u=(1.6* 6.26418)/(0.01)\\\Rightarrow u=1002.2688\ m/s`

The magnitude of velocity just before hitting the block is 1002.2688 m/s