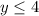Question

Which function has a range of vy s 5)?

of(x) = (x - 4)2 + 5
O (x) = -(x - 4)2 + 5
o f(x) = (x - 5)2 + 4
Of(x) = -(x - 5)2 + 4

Answer 1

see the explanation

Explanation:

Verify the range of each quadratic function

case 1) we have

`f(x)=(x-4)^2+5`

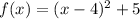
This is a vertical parabola open upward (the leading coefficient is positive)

The function is written in vertex form

The vertex represent a minimum

The vertex is the point (4,5)

The range is the interval [5,∞)

`y\geq 5`

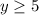
case 2) we have

`f(x)=-(x-4)^2+5`

This is a vertical parabola open downward (the leading coefficient is negative)

The function is written in vertex form

The vertex represent a maximum

The vertex is the point (4,5)

The range is the interval (-∞,5]

`y\leq 5`

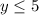
case 3) we have

`f(x)=(x-5)^2+4`

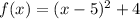
This is a vertical parabola open upward (the leading coefficient is positive)

The function is written in vertex form

The vertex represent a minimum

The vertex is the point (5,4)

The range is the interval [4,∞)

`y\geq 4`

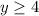
case 4) we have

`f(x)=-(x-5)^2+4`

This is a vertical parabola open downward (the leading coefficient is negative)

The function is written in vertex form

The vertex represent a maximum

The vertex is the point (5,4)

The range is the interval (-∞,4]

`y\leq 4`