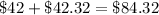Answer:
Part a)

Part b)
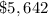
Part c)

Part d)
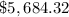
Part e)
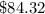
Explanation:
The complete question is
Rhonda deposits $5,600 in a savings account that pays 1.5% interest, compounded semi-annually. Round to the nearest cent.
a. How much interest does the account earn in the first 6 months?
b. What is the ending balance after 6 months?
c. How much interest does the account earn in the second 6 months?
d. What is the balance after 1 year?
e. How much interest does the account earn the first year?
we know that
The compound interest formula is equal to
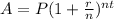
where
A is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
n is the number of times interest is compounded per year
Part a) How much interest does the account earn in the first 6 months?
in this part we have

substitute in the formula above

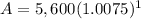
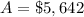
Find out the interest
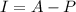
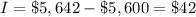
Part b) What is the ending balance after 6 months?
we know that
The ending balance after 6 months is the same that the final investment value of A after 6 months
so
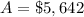 ----> see part a)
----> see part a)
Part c) How much interest does the account earn in the second 6 months?
in this part we have

substitute in the formula above
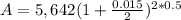
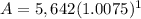
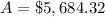
Find out the interest
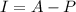

Part d) What is the balance after 1 year?
we know that
The balance after 1 year is equal to the initial deposit of $5,600 plus the interest earned in the first 6 months plus the interest earned in the second 6 months
so
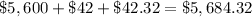
Part e) How much interest does the account earn the first year?
The total interest the first year is equal to the interest earned in the first 6 months plus the interest earned in the second 6 months
so