Question

N a simple random sample of 219 students at a college, 73 reported that they have at least $1000 of credit card debt.

Which interval is the 99% confidence interval for the percent of all the students at that college who have at least $1000 in credit card debt?



(31.0 ,35.6)



( 30.1 , 36.5)



(25.0 ,41.6)



(27.5 ,39.1 )

Answer 1

`0.333 - 2.58 \sqrt{(0.333(1-0.333))/(219)}=0.251`

`0.333 + 2.58 \sqrt{(0.333(1-0.333))/(219)}=0.416`

The 99% confidence interval would be given (0.251;0.416).

(25.0 ,41.6)

Explanation:

1) Data given and notation

n=219 represent the random sample taken

X=73 represent the students that reported that they have at least $1000 of credit card debt.

`\hat p=(73)/(219)=0.333` estimated proportion of students that reported that they have at least $1000 of credit card debt.

`\alpha=0.01` represent the significance level

z would represent the statistic (variable of interest)

p= population proportion of students that reported that they have at least $1000 of credit card debt.

2) Confidence interval

The confidence interval would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

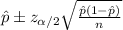
For the 99% confidence interval the value of
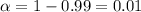`\alpha=1-0.99=0.01` and
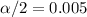`\alpha/2=0.005`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=2.58`

And replacing into the confidence interval formula we got:

`0.333 - 2.58 \sqrt{(0.333(1-0.333))/(219)}=0.251`

`0.333 + 2.58 \sqrt{(0.333(1-0.333))/(219)}=0.416`

And the 99% confidence interval would be given (0.251;0.416).

We are confident that about 25.1% to 41.6% of students have at least $1000 of credit card debt.

And for this case the most accurate option is:

(25.0 ,41.6)