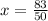Question

Given points A (1, 2/3), B (x, -4/5), and C (-1/2, 4) determine the value of x such that all three points are collinear

Answer 1

`x=(83)/(50)`

Explanation:

we know that

If the three points are collinear

then

`m_A_B=m_A_C`

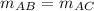
we have

A (1, 2/3), B (x, -4/5), and C (-1/2, 4)

The formula to calculate the slope between two points is equal to

`m=(y2-y1)/(x2-x1)`

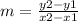
step 1

Find the slope AB

we have

`A(1,(2)/(3)),B(x,-(4)/(5))`

substitute in the formula

`m_A_B=(-(4)/(5)-(2)/(3))/(x-1)`

`m_A_B=((-12-10)/(15))/(x-1)`

`m_A_B=-(22)/(15(x-1))`

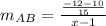
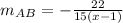
step 2

Find the slope AC

we have

`A(1,(2)/(3)),C(-(1)/(2),4)`

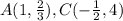
substitute in the formula

`m_A_C=(4-(2)/(3))/(-(1)/(2)-1)`

`m_A_C=((10)/(3))/(-(3)/(2))`

`m_A_C=-(20)/(9)`

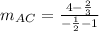
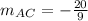
step 3

Equate the slopes

`m_A_B=m_A_C`

`-(22)/(15(x-1))=-(20)/(9)`

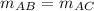
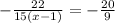
solve for x

`15(x-1)20=22(9)`

`300x-300=198`

`300x=198+300`

`300x=498`

`x=(498)/(300)`

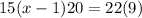
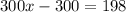
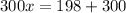
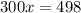
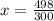
simplify

`x=(83)/(50)`