Answer:
Perimeter of ΔABC:
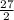 +
+
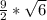 units
units
Area of ΔABC:
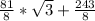 units
units
Skills required: HS Geo, Special Triangles
Explanation:
1) The best option is to break down this triangle. Let's draw an altitude from Point B down to Segment AC. The point from the altitude that intersects AC is Point D. BD is the height of our triangle, AC is the base.
2) Angle A is 60 degrees, and since Angle BDA is 90 degrees, Angle ABD is 30 degrees. We can use the 30-60-90 degree right triangle property for the triangle BDA.
- This states that if the side opposite the 30 degree angle is
 , the side opposite the 60 degree angle is
, the side opposite the 60 degree angle is
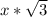 , and the side opposite the 90 degree angle is
, and the side opposite the 90 degree angle is
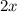 .
.
AB is 9 units, and it is opposite the 90 degree angle. This means that
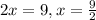 ==> This then means that AD, the segment opposite the 30 degree angle in this triangle is
==> This then means that AD, the segment opposite the 30 degree angle in this triangle is
 units. Segment BD (the height) is
units. Segment BD (the height) is
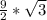 .
.
3) Angle C is 45 degrees, and Angle BDC is 90 degrees, which means that Angle CBD is 45 degrees. We can use the 45-45-90 degree right triangle property for the triangle BCD.
- This states that if the side opposite the 45 degree angle is
 , the other side opposite a 45 degree angle is also
, the other side opposite a 45 degree angle is also
 , but the hypotenuse (side opposite the right (90 degree) angle) is
, but the hypotenuse (side opposite the right (90 degree) angle) is
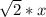 .
.
BD is
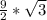 , which means DC is the same. BC, which is the hypotenuse is BD multiplied by square-root-2, which is
, which means DC is the same. BC, which is the hypotenuse is BD multiplied by square-root-2, which is
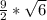 .
.
4) Area is
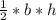 , the base (b) is AC (which is
, the base (b) is AC (which is
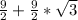 ), the height is BD (
), the height is BD (
 ). When multiple you will get
). When multiple you will get
 , then this multiplied by 1/2 is
, then this multiplied by 1/2 is
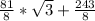 <--> this is the area!
<--> this is the area!
5) Perimeter is just the sum of all side: 9 +
 +
+
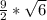 =
=
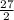 +
+
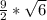 unit
unit