Part A
Each triangle shown is a 45-45-90 triangle. The numbers refer to the angle measures. These types of triangles are known as isosceles right triangles.
The two legs of these triangles are the same length. So for this specific triangle, the two legs are 28 units each.
The hypotenuse can be found using the pythagorean theorem
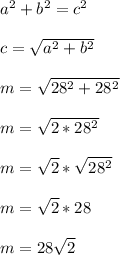
We can avoid using the pythagorean theorem by remembering the rule that the hypotenuse is exactly sqrt(2) times that of either leg length.
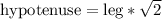
This shortcut only applies to 45-45-90 triangles.
Answer:
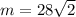
=============================================================
Part B
Again we can use the pythagorean theorem to find the missing variable.
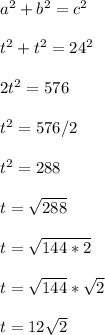
Or we could follow the steps like this

The second set of steps only apply for 45-45-90 triangles.
Answer:
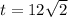
=============================================================
Part C
Like mentioned earlier, the two leg lengths are the same.
Therefore,
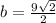
=============================================================
Part D
Multiply the leg lengths by the factor sqrt(2) to determine the hypotenuse
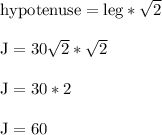
Or you could use the pythagorean theorem as an alternative route.