Answer:
a₆ = - 486
Explanation:
The nth term of a geometric sequence is
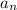 = a₁
= a₁
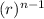
where a₁ is the first term and r the common ratio
Here a₁ = - 2 and r = 3 , then
a₆ = - 2
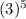 = - 2 × 243 = - 486
= - 2 × 243 = - 486
The recursive rule allows a term in the sequence to be found by multiplying the previous term by the common ratio, that is
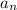 = 3
= 3
 with a₁ = - 2
with a₁ = - 2