Answer:
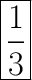
Explanation:
This question requires you to make common denominators. A fraction, similarly to an equation, can have operations applied to them, as long as that operation affects both the numerator and denominator.
Because the LCM, or Lowest Common Multiple, of 5, 15, and 3 is 15, simply make the denominator of every fraction 15.
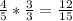
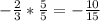
Now, simply substitute the new fractions to get:

Then, simplify the fraction to get

Hope it helps :) and let me know if you want me to elaborate or provide alternate methods.