Question

An equilateral triangle has sides that measure 5x+3 and 7x-3 feet. x=

Answer 1

x = 3

Explanation:

equilateral means the sides are the same:

5x+3 = 7x-3

Answer 2

x = 3

Explanation:

Given an equilateral triangle whose two sides have measures of m∠(5x + 3)° and m∠(7x - 3)°.

We can establish the following equality statement since both sides have the same measure:

5x + 3 = 7x - 3

To start with, add 3 to both sides of the equation:

5x + 3 + 3 = 7x - 3 + 3

5x + 6 = 7x

Next, subtract 5x from both sides:

5x -5x + 6 = 7x - 5x

6 = 2x

Divide both sides by 2 to solve for x:

`\mathsf{(6)/(2) = (2x)/(2)}`

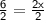
x = 3

Therefore, the value of x = 3.

Substitute the value of x into the equality statement to find the measures of the sides of the given triangle:

5x + 3 = 7x - 3

5(3) + 3 = 7(3) - 3

15 + 3 = 21 - 3

18 = 18 (True statement).

Thus, the measure of each side of the triangle is 18, making the value of x = 3 as a valid answer.