Explanation:
Before I discuss how to graph both equations, I believe that it is essential to understand the variables and constant related to linear equations.
The slope-intercept form is: y = mx + b, where:
m = slope (which tells you the steepness of the line).
b = y-intercept: this is the point on the graph where it crosses the y-axis. Its coordinates is always formatted as, (0, b). The b is the y-coordinate of the y-intercept that you use for the equation.
The standard form of linear equations is: Ax + By = C, where:
A, B, C are integers (whole numbers, and cannot be in fraction or decimal forms). A and B ≠ 0, and A must be a positive integer.
Now that I have defined what the slope-intercept and standard form of linear equations are, we can proceed with working on graphing both equations.
7. y = -6x - 4
In this equation, the slope (m) = -6, and the y-intercept (b) = -4. As an ordered pair, the y-intercept is (0, -4). In order to graph this equation, start by plotting the y-intercept on the graph. Then, use the slope (rise over run) to plot other points on the graph (6 units down, 1 unit run). Your next point should occur at (1, -10).
You could simply connect these two points and draw a line, or plot another point on the graph using the slope technique mentioned in this post.
8. 3x - 4y = 12
This equation is in standard form, Ax + By = C. It is easier to graph a linear equation when it is in its slope-intercept form, y = mx + b.
In order to transform this equation into its slope-intercept form, start by subtracting 3x from both sides of the equation:
3x - 4y = 12
3x - 3x - 4y = - 3x + 12
-4y = -3x + 12
Next, divide both sides by -4 to isolate y:
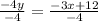
y = ¾x - 3 ⇒ This is the slope-intercept form where the slope, m = ¾, and the y-intercept is b = -3.
Similar to how we graphed the equation from question 7, start by plotting the y-intercept, (0, -3). Then, use the slope of the equation, m = ¾ (3 units rise, 4 units run) to plot the next point. The next point should occur at (0, 4).
Attached are the graphed equations.