Question

A toy car of mass 2-kg travels along a horizontal surface with negligible friction at a speed of 1.0 m/s. The car then collides with a vertical wall. The wall applies a force of magnitude 20 N for 0.2 s on the toy car. Which of the following predicts the motion of the toy care immediately after the collision?

A: The speed of the car will increase, and the car will travel in the opposite direction.
B: The speed of the car will decrease, and the car will travel in the opposite direction.
C: The speed of the car will remain the same, and the car will travel in the opposite direction.
D: The car will stop and remain motionless.

Please show your work in finding the direction of motion - ie. please don't use common sense but rather show the applicable physics principles along with formulas to find the direction of motion.

Answer 1

• Mass=m=2kg
• Velocity=v=1m/s
• Momentum=mv=2kgm/s

As we know that

• Force=dp/dt(Change in momentum/time)

So

• 20N=dp/2
• dp=10N/s

• Hence the speed of car will remain the same it won't affect the speed
• As momentum from wall is more the ball will travel in opposite direction

Option C is correct

Answer 2

The final velocity (
`\(v_{\text{final}}\)`) is positive, indicating that the car continues to move in the same direction after the collision. Therefore, option A is the correct prediction.

To determine the motion of the toy car immediately after the collision, we can apply the principles of linear momentum. The conservation of linear momentum states that the total linear momentum of an isolated system remains constant if no external forces act on it.

The initial linear momentum (
`\(p_{\text{initial}}\)`) of the system is given by the mass of the car (m) multiplied by its initial velocity (
`\(v_{\text{initial}}\)`):

`\[ p_{\text{initial}} = m \cdot v_{\text{initial}} \]`

The final linear momentum (
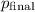`\(p_{\text{final}}\)`) of the system is given by the mass of the car multiplied by its final velocity (
`\(v_{\text{final}}\)`):

`\[ p_{\text{final}} = m \cdot v_{\text{final}} \]`

Since no external horizontal forces act on the system, the horizontal component of linear momentum is conserved. Mathematically, this can be expressed as:

`\[ p_{\text{initial, horizontal}} = p_{\text{final, horizontal}} \]`

Now, let's break down the velocities into components. The initial velocity (
`\(v_{\text{initial}}\)`) is positive since the car is moving to the right. After the collision, the final velocity (
`\(v_{\text{final}}\)`) will have both magnitude and direction.

Given that a force is applied in the opposite direction, we can use the impulse-momentum theorem:

`\[ \text{Impulse} = F \cdot \Delta t \]`

The change in momentum (
`\(\Delta p\)`) is equal to the impulse:

`\[ \Delta p = F \cdot \Delta t \]`

Now, the final velocity (
`\(v_{\text{final}}\)`) can be calculated using the formula:

`\[ v_{\text{final}} = v_{\text{initial}} + (\Delta p)/(m) \]`

Substituting the values:

`\[ v_{\text{final}} = 1.0 \, \text{m/s} + \frac{(20 \, \text{N} \cdot 0.2 \, \text{s})}{2 \, \text{kg}} \]`

`\[ v_{\text{final}} = 1.0 \, \text{m/s} + 2.0 \, \text{m/s} = 3.0 \, \text{m/s} \]`

The final velocity (
`\(v_{\text{final}}\)`) is positive, indicating that the car continues to move in the same direction after the collision. Therefore, option A is the correct prediction:

A: The speed of the car will increase, and the car will travel in the opposite direction.