Question

Use the substitution method to solve the following system of equations:

3x + 5y = 3
x + 2y = 0

Answer 1

`\displaystyle [6, -3]`

Step-by-step Step-by-step explanation:

To begin this process, we will first select the second equation. Here is how the Substitution method wourks:

`\displaystyle \left \{ {{3x + 5y = 3} \atop {x + 2y = 0}} \right. \\ \\ \\ \left \{ {{y = 2x + 3} \atop {x = -2y}} \right. \\ \\ 3[-2y] + 5y = 3 \hookrightarrow -6y + 5y = 3 \hookrightarrow -y = 3; -3 = y, 6 = x \\ \\ \\ \boxed{[6, -3]}`

So, you substitute
`\displaystyle x`for
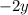`\displaystyle -2y`and continue combining like-terms until you receive the y-value. You then plug this back into the system to get the x-value, which was what you saw.

I am joyous to assist you at any time.