Question

NO LINKS!! A blimp, suspended in the air of 500 feet, lies directly over a line from Soldier Field to the Adler Planetarium on Lake Michigan (see the figure). If the angle of depression from the blimp to the stadium is 32° and from the blimp to the Planetarium is 23°, find the distance between Soldier Field and the Adler Planetarium.

​

Answer 1

1978 ft (nearest foot)

Explanation:

Using the Alternate Interior Angles theorem, we can deduce that the left base angle of the triangle is 32° and the right base angle of the triangle is 23°.

The triangle can be divided into 2 right triangles, both with height of 500ft (see attached diagram).

Using the tan trig ratio, we can calculate the base of each triangle.

`\mathsf{\tan(\theta)=(O)/(A)}`

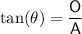
where:

• 
  `\theta` = angle
• O = side opposite the angle
• A = side adjacent the angle

Left triangle

let
`x` = the base length

`\implies \tan(32)=(500)/(x)`

`\implies x=(500)/(\tan(32))`

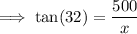
Right triangle

let
`y` = the base length

`\implies \tan(23)=(500)/(y)`

`\implies y=(500)/(\tan(23))`

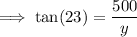
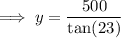
To find the distance between the Soldier Field and Adler Planetarium, simply sum
`x` and
`y`:

`\implies (500)/(\tan(32))+(500)/(\tan(23))=1978.093447...`

Therefore, the distance is 1978 ft (nearest foot)