Answer:
Explanation:
A convergent series is a series whose partial sums tend to a specific number or a limit.
Example:
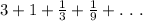
This is because a series is convergent if
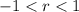
where r is the common ratio
and for the question above
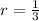
A divergent series is a series whose partial sums do not approach a limit. Divergent series typically go to positive infinity, negative infinity, or don't approach a specific number.
Example:
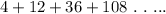
This is a divergent series because the common ratio is;

Hope this helps! =)
If you have any queries please ask.