Consider the given quadratic equation that we have :
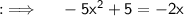
Multiplying both sides by -1 will yield ;
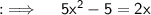
Write the above quadratic equation in the form of standard quadratic equation ax² + bx + c = 0 ,
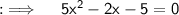
Now , comparing this with the standard form of quadratic equation we will get a = 5 , b = -2 , c = -5 . So now , Discriminant (D) = (-2)² - 4 × 5 × -5 = 4 + 100 = 104
Now , by the quadratic formula ;
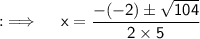
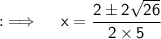
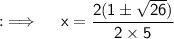
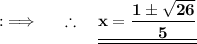
Used Concepts :-
For any quadratic equation of the form ax² + bx + c , the Discriminant (D) is given by D = b² - 4ac , and the root
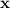 of the quadratic equation is given by the quadratic formula as
of the quadratic equation is given by the quadratic formula as