You have a compound inequality,

which is the same as saying
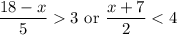
(the
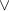 symbol means "or") which in plain English translates to
symbol means "or") which in plain English translates to
"x belongs to some set of numbers such that the quantity (18 - x)/5 is larger than 3, or the quantity (x + 7)/2 is smaller than 4"
Solve the two inequalities separately. On their own, there are no hidden tricks to solving them.
(18 - x)/5 > 3
Multiply both sides by 5 :
18 - x > 15
Isolate x :
18 - 15 > x
x < 3
(x + 7)/2 < 4
Multiply both sides by 2 :
x + 7 < 8
Isolate x :
x < 8 - 7
x < 1
So the solution to the compound inequality is x < 3 or x < 1, meaning "the set of real numbers x that are either smaller than 3 or smaller than 1". But if x < 1, then x is automatically smaller than 3, so the "or" solution can be condensed into a single inequality, x < 3.
The thing about "or" statements in math is that the compound condition, "(condition 1) or (condition 2)", is met if at least one of condition 1 or condition 2 is met.